The Precession of the Equinox of the Earth is associated with another celestial phenomenon: Perihelion Precession - which is typically considered in the context of Mercury's orbit. Be careful not to conflate these two concepts, they are very different.

We are told that observation of Mercury's perihelion advances by 5600 arc-seconds per century. And we are also told that Mercury's perihelion physically advances by about 545 arc-seconds per century. Those differences in amounts of Perihelion Precession are said to be reconciled by the Precession of the Earth's Equinoxes, as well as Einstein's Relativity.

How exactly does 'classical theory' use as reference the value of 5600, when the amount that Mercury's perihelion is preceding is actually about 10 times less? Miles Mathis outlines these details succinctly:

Let us just get a good definition of what Godoi and Mathis tell us about 'Precession of the Equinoxes'. Bear in mind that this concept 'Precession of the Equinoxes' is said to advance observation of Mercury's perihelion by 10 times more than the effect of all the gravity of the other planets put together.

So the direction in which the Earth's axis is pointing, is itself rotating. Precession of the Equinox is also termed: Axial Precession. We all know that the Earth spins on it axis. But the movement of the Earth's axis itself describes a cycle equivalent to the Arctic circle (center diagram below). Wikipedia rounds it off quite bluntly (quoted 2018), but nevertheless they depict it quite nicely:

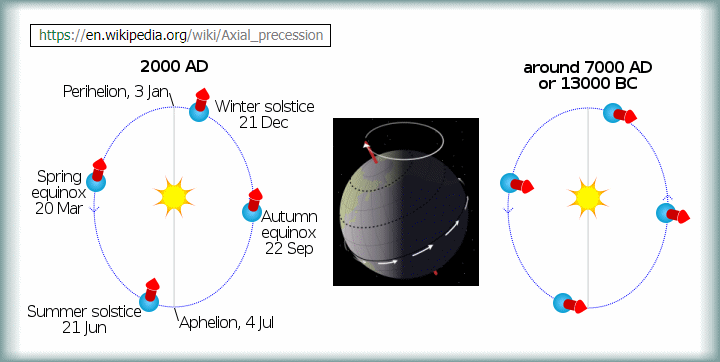
Because there are 1.296 million arc-seconds in any cycle,
a period of 25772 years will yield 1296000/25772 = 50.287 arc-seconds per year.
The precession of the Earth's equinox is then given as 5029 arc-seconds per century.

How does that terrestrial event equate to Mercury's orbit?
In two different ways:

If we adjust for the effect on our spatial position, then we only get an amount of 0.1 arc-seconds per century that Axial Precession changes our perspective of Mercury. But it distorts our temporal perception of the length of a year more profoundly.

This is because the time it takes for the Earth to orbit the Sun is: 365.256 days - a sidereal year, which is not the time it takes between a pair of winter solstices: 365.242 days - the tropical year.

If we mistakenly use the tropical year, instead of the sidereal year then we observe the orbit of Mercury (or any other planet), with an error of 5029 arc-seconds per century. This 50.29 arc-seconds per year is then also exactly the difference between the sidereal and tropical year, which equates to about 20 minutes of time.

If you are constructing an n-body algorithm of the solar system then you must pay attention to this, and the section later where I explain all of this in more detail: Sidereal Year or Tropical Year. But the 5029 arc-second per century amount is an error that needs to be understood as a matter of rigorous methodology.

In fact, most others have been able to skip over this detail completely, and simply calculate Mercury's Perihelion Precession using the proper sidereal year. And there certainly is much utility in this route. But in doing this, they would have accepted the notion that the other planets can cause the Earth's axis to wobble every 26000 years or so.

I shall put this aside for now, and just take the precise and shorter route of what is the correct way to measure Mercury's Perihelion Precession according to sidereal years; which properly then avoids the Precession of the Equinox and its incorrect yield from tropical years.

In order to begin our understanding of Mercury's Perihelion Precession, we need to be clear that the most vital step in understanding n-body-gravity is to check just what Newton's theory actually dictates in an evolutionary 3d-algorithm, before we can go beyond this to the 'observations' and other theories on gravity.

We need to be methodical in our approach. So our starting point should be the interaction between Jupiter and Saturn as they have the greatest influence on the entire solar system; and they constantly alter one another. So if their dynamic is not properly understood then the entire process is going to be flawed.

If it is not clear to the reader how Jupiter and Saturn alter orbital structure, then look at the section 'Jupiter+Saturn' before getting back to what follows. The next section is a proper calculation of Perihelion Precession for Mercury according to Newton's law with the correct measurement only in sidereal years. I cannot quite make up my mind which section should be read next myself. You may want to divert to more detail of Sidereal Year or Tropical Year.

<— Page Back — Start Page — Next Page —>

see also: — Jupiter+Saturn — Sidereal Year or Tropical Year