The purpose of this article is to determine
the velocity at which the force of gravity is propagated.

The central finding of this computational analysis is that whilst the LIGO GW150914 gravitational-wave experiment is a vital component of astrophysics theory from an engineering and experimental perspective, the theoretical findings in article PhysRevLett.116.061102 (hereafter referred to as Abbot) are intrinsically flawed when programmed in an evolutionary computer algorithm.

The software used in this analysis is free here: Download orbit-gravity-sim-11.exe.
See help files on how orbit-gravity-sim-11.exe works here: OGS11 simulator physics.

These 10 html pages are summaries of the full analysis which can be downloaded here:
download: Chapter 29 - Computational Analysis of LIGO GW150914
.

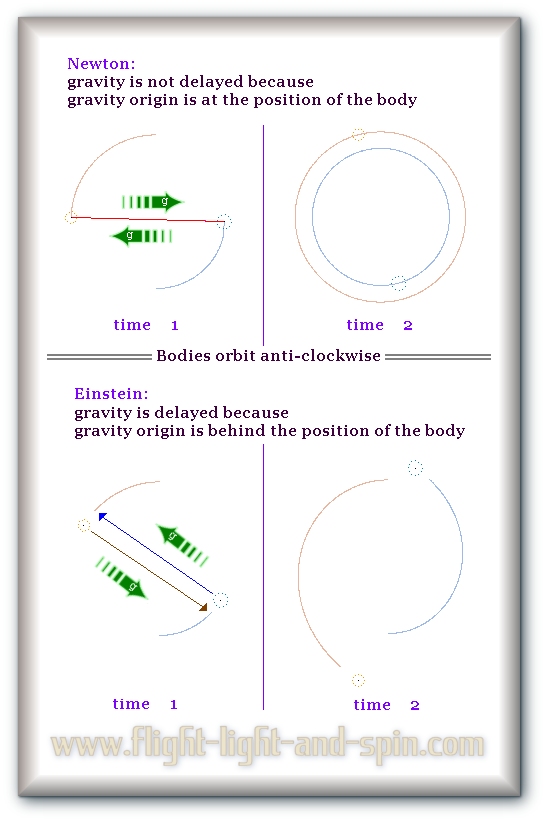
Abbot inherits the claim by Einstein that the gravitational-wave theory (gravity propagating at the velocity of light) will result in binary celestial bodies spiraling inwards towards the center of the system.

The main reason why this article claims that both Einstein & Abbot's theoretical analysis is incorrect is simply termed: BOGVOS - Binary-Orbit, Gravity-Velocity, Out-Spiral.

In the simulated computational graphics above, each of the systems evolves differently according to the two theories on the velocity of gravity at two different non-specified times (time 1 and time 2).

The Newtonian orbits return to their origin due to them pulling perpendicular to the movement of the body.

However, with parameters similar to the LIGO pair including a delay in gravity caused by the velocity of gravity being the same as the velocity of light; the pair out-spirals so quickly that it is easy to observe.
.

These web-pages are a brief summary of the full article.
.

pg		Summaries of Sections
1		Introduction to the Analysis
2		The Source of Gravitational Energy
3		The Shape of the LIGO Waveform
4		The Cause of the Binary In-spiral
5		Gravity Velocity and Binary Orbits
6		White Dwarf Binary Gravity Velocity
7		Conclusions & Beginnings
8		Using OGS11 Gravity Simulator
9		What is GW150914 actually?
10		Download orbit-gravity-sim-11.exe
^ Top of Page ^

.