The simplest math of music theory.

The basic harmony between a pair of notes one 'octave' apart, is said to be the simplest and most harmonious. A1 and A2 resonate because the frequency is 'precisely' such that:
A2 is double the frequency of A1. (2:1)

A1 = 55Hz.    A2 = 110Hz.
Same with any other pair of such notes: B2 = B1 x 2, etc...

Our ears find pleasant that perfect and very simple mathematical relationship in doubling or halving the waveform. This itself is amazing; and if you think on it deeply, this is actually proof of Plato's forms. (Contrarian vexation aside).

The next best harmony is what music theory sometimes calls the 'fifth'. The note A resonates with D at 'precisely' the wavelength / frequency relationship of 4:3. How incredible!

The two notes A & D are 5 notes apart, that is why I call it a 'fifth'.
The other simplest ratio produces another harmonious sound.
We can also count in 7ths and get the frequency ratio 3:2

Of course, counting 5 up or 7 down will get you the same note.
And counting 7 up and 5 down will also get the same note.
Because there are 12 notes in the full chromatic scale.

It does not matter which note you start with.
If the note that is 5 or 7 steps away is played they will sound harmonious together.

Now often I read that a 'fifth' is 7 notes apart.
Of course that is convoluted. It all depends whether you are counting up or down!

Just because most websites or people say something does not make it true, most people just copy-paste. Count the number of notes yourself!

Does it make sense to say that a fifth is 7 notes apart?
or does it make sense to say that a fifth is 5 notes apart?

Why do I use the word 'precisely' in quotes?
Because in music theory, the wavelengths and/or frequencies are not quite at those perfect ratios! In the math of physics, they are precise! That is why I continually say that music theory is based on faulty math. Let me now prove this to you with pure logic.

If we start with A1, and then jump 60 notes up,
we have moved 12 jumps of five, but also 5 jumps of twelve.
That is, 5 octaves. This is simply because 5 x 12 = 60

{Why do we use the word octave (meaning 8) when we really mean 12?
Because music theorists are bad at math!
The word 'octave' is a misnomer based on how pianos are built}

Nevertheless, let us look at the 60 note progression.
If we note the frequency of A1 at 55Hz, then
we get this progression: A1 x 2 = A2 which is 110Hz.
A3=220, A4=440, A5=880, A6=1760 which is 5 octaves.
That is great and fine and simple.

It is very easy to see that 2 x 2 x 2 x 2 x 2 = 32
and thus 32 x 55Hz = 1760 Hz, which is the note of A6.

But!

If we do a similar progression with jumps of 5 notes (12 of them), then it looks like this:
(4/3)x(4/3)x(4/3)x(4/3)x(4/3)x(4/3)x(4/3)x(4/3)x(4/3)x(4/3)x(4/3)x(4/3)
= 31.5692918~

It should be clear that 31.57~ is not the same as 32.
So when we multiply by our starting 55Hz, now we get:

A6 = 1736.311 Hz...

which is not the same as 1760 Hz in our simpler example above.
That is quite a big problem.

So what instrument-makers and software-developers both do, is to take that tiny error and fudge (or distribute) it over the whole spectrum of sounds, and thus there is always imperfection in our musical system.

It is said to be too small to be really all that noticeable. But is it? The more octaves you are trying to reconcile, the more fudging needs to be done, so that your highest and lowest notes are in harmonious agreement.

So why is this important to making music?
Why should you care?

Well, if you play a pair of notes 5 octaves apart, you will typically get dissonance when you expect resonance according to music theory! And that depends on how the fudge is spread.

So far we have only described pairs of notes. When you have several notes, the frequency ratios become painfully impossible to calculate.

But!

All you really need to know is that a pair of notes that take a double jump of five notes apart are also simply two notes apart. Think a bit. How is double 5 equal to 2?
Because 2 fifths is 10 notes.
And 12 - 10 = 2

So long as you appreciate that an octave jump has occurred, then 2 jumps of 5, is the same as one jump of 2. So if I jump up from A to D, (5), then again from that D to G, (another 5) we can see that G is 2 away from the start which was A.

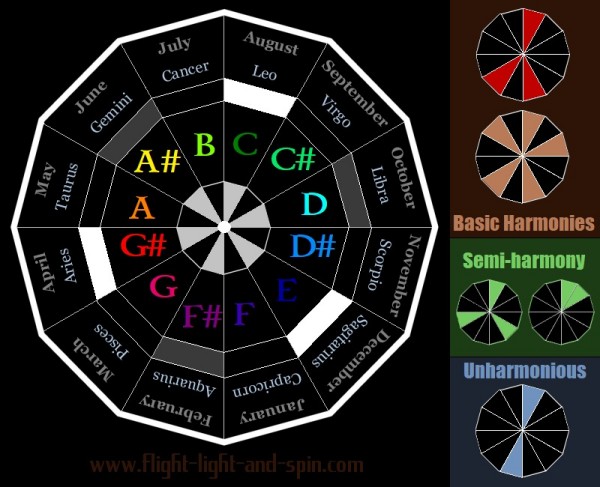
So we now know that harmonies are in jumps of 5, 7, and 2. But!
If a jump of 2 is good and a jump of 5 is good, then it follows that a jump of 3 is also good!
You can see this visually in the diagrams towards the end of this page.

So we really only have jumps of 2, 3 & 5.
This is because 7 is effectively virtually the same relationship as 5.
Very crudely put: Prime numbers good. Non-primes bad.

And that is all you really need to know about music theory.

When it comes to the more complex arrangements, you have to rely on your ear, because the math just gets ridiculous in itself, nevermind the fudge that confuses it even more!

But if you start with basic building blocks of 2 and 5 you will have a good foundation from which to build those more complex sounds. You can build simple melodies very quickly, without even needing to hear the music all that much. You can rapidly calculate a solid foundation. Then you can spend most of your time working on the detail of the intricate melody, using your ear. Trial and error is vital, but is much more effective if you have a solid foundation.

Of course, lyrics are another matter entirely; and the way words resonate with melodies is as much mysticism as it is psychology...