| Volume of the Universe The shape of the Universe has been established as a 3d-hyper-surface of a 4d-hyper-sphere. But if we are dealing with a 1d-Universe on the edge of a 2d-disc, or instead of this we also consider flatland: a 2d-Universe on the surface of a 3d-sphere; then the ratio between the diameter and the circumference is still pi. So it stands to reason that this same ratio of pi is still perfectly good for a 4d-hypersphere. However
when we look back to the origin of the Universe, we are not looking
back directly along the radius. We are looking outwards, and then
back. Consider this diagram very slowly and carefully: |
||
|
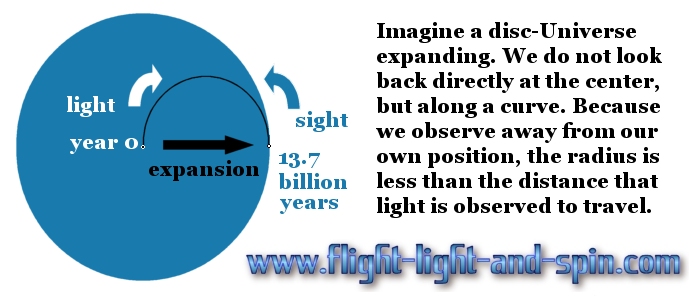
The inhabitants of a disc-Universe live on the 1-dimensional edge.
If the light could travel directly along the radius of expansion,
they would see themselves in the past. But instead they see objects
a distance away from them on the expanding edge. So the light cannot
travel directly from the centre to the edge along the radius of expansion.
This
may be difficult to comprehend at first, but once you see it, then
it should be fairly easy to conclude from this premise that: The
radius is thus 13.7 billion light-years divided by half of pi: Calculating
the volume is a bit trickier because we need the formula for the
3d-hyper-surface of a 4d-hyper-sphere. Of course the 4d-hyper-volume
is not the same as this because that would be a measurement in m^4,
whereas a 3d measurement results in m^3. |
||
|
This is also not the same as a simple 3d-volume either because our
Universe has no visible 3d-edge to it like all 3d-volumes do actually
have.
So
the volume of the Universe is 1.1x10^79
m^3 or 1.1x10^70
km^3: |
||
|
Although, some would claim that 4d-space is an untenable idea. So
is it meaningful to postulate the possibility that space is just
ordinary 3d-space? In this sense, the galaxies would all be receding
from our current position into an endless void. In this scenario,
all the mass in the Universe (3x10^52kg)
could have at some point been contained in a small spherical volume
of space smaller than the distance to the nearest star. At this
point it would have had the average density of water. But then the
escape velocity would have been 70 000 times the velocity of light.
The obvious problem here is that such a theory would not be able
to allow for the Relativistic notion that velocity cannot exceed
the velocity of light.
But
what would the radius of the mass of the Universe need to be,
such that its escape velocity is equal to the velocity of light? |
||
 |
||
|
And it is quite a surprise to find that the mass of the Universe
would need to be 4.7 billion light-years in radius to have an escape
velocity of the speed of light. If you are a die-hard Relativist
may need to think about that in the context of how you have conceptualized
the Universe when it was less than 4.7 billion-light-years in radius. . |
||
| This is an extract summary of Chapter XXX of the book: Flight Light and Spin Download page for relativity simulation: algorithm orbit-gravity-sim-12.exe The full chapter can be downloaded here: Sum-Theory.pdf (5.5 mb, 57 pages, this pdf file is too big for chrome, use firefox) List of: abbreviated short articles . . |
|||||